Non-uniform Scale of Electrodynamometer Type Instrument

Unlike a PMMC instrument, the electrodynamometer type instrument has non-uniform scale. The non-uniform scale of this instrument can be explained by the equation of deflection. As we know that the deflection in electrodynamometer type ammeter and voltmeter is given as

O = KI²dM/dO for ammeter

and O = KV²dM/dO for voltmeter

Therefore, if dM/dO is assumed constant then clearly the deflection of needle follows purely square law and therefore the scale will also follow the square law. But actually dM/dO is not constant rather it varies with the movement of moving coil. It is suggested to read the construction of Electrodynamometer type instrument for better understanding.

Why non-uniform Scale of Electrodynamometer Type Instrument?

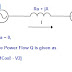
Actually, the value of dM/dO is constant for radial magnetic field but as in electrodynamometer type instrument the magnetic field is parallel therefore dM/dO is not constant. Figure below shows this parallel field produced by the fixed coil of instrument.

Actually, the mutual inductance M between the fixed coil and moving coil depends on the position of the moving coil. As can be seen from figure, the maximum value of mutual inductance M_max will take place when the plane of moving coil is perpendicular to the line of field i.e. O = 180�. Don�t get confused that, we are taking of perpendicular but still saying that O = 180�. Actually, to have maximum mutual inductance the flux linkage in the moving coil shall be maximum and this will take place when the plane of the moving coil is perpendicular to the field. If we put O = 180�, we observe that, the flux linkage in moving coil is maximum. Even if we put O = 0, then also the mutual inductance will be maximum but we are assuming flux linkage to be maximum positive when O = 180�. Therefore at O = 0, the mutual inductance will be maximum negative.

Using this concept, we can say that the mutual inductance M is varying sinusoidally with the movement of moving coil and therefore it can be written as

M = -M_maxCosO

Hehehe�you will think that why didn�t we wrote it as M = M_maxSinO? Actually we need to get dM/dO, therefore if M = -M_maxCosO then dM/dO = M_maxSinO which is more usable for us. By the way, you can consider M = M_maxSinO and do rest of the calculations based on this.

dM/dO = M_maxSinO

Therefore deflecting torque of dynamometer type instrument,

T_d = I₁I₂M_maxCos�SinO

But Td = KO

So deflection

O = (I₁I₂M_maxCos�SinO)/K

From the above equation of deflection, it is clear that instrument do not have purely square law response. It is also clear from the above equation that electrodynamometer type instrument has non-uniform scale.

But in practical, the instrument is constructed such that O varies from -45� to +45� from the position of zero mutual inductance. The change in M over this range is not much large and for all practical calculation, this change in M i.e. dM/dO can be considered constant. Thus an electrodynamometer type instrument available in market has almost square response.