Torque Equation in Electrodynamometer Instruments

Electrodynamometer Instruments are widely used as an ammeter, voltmeter and wattmeter. This instrument can measure AC as well as DC quantities. This means that the torque in electrodynamometer instruments must have a finite value and unidirectional for AC and DC quantity unlike PMMC instruments. In this post we will be discussing the torque equation in such instruments.

Torque Equation in Electrodynamometer Instruments

Electrodynamometer  instruments or Electrodynamic instruments have fixed coil divided into two sections and a moving coil. It is recommended to read before going to understand the torque equation. If you are already aware of constructional feature then you may proceed.

Let us assume that the current in fixed coil be I₁ and that in moving coil be I₂ as shown in figure below.

Also assume that,

L₁ = Self inductance of fixed coil

L₂ = Self inductance of moving coil

M = Mutual inductance between fixed and moving coils

Thus,

The flux linkage of fixed coil �₁ = L₁i₁ + Mi₂

The flux linkage of moving coil �₂ = L₂i₂ + Mi₁

The electrical energy input to the instrument,

= e₁i₁dt + e₂i₂dt

But according to Faraday�s Law,

e₁ = d �₁/dt

and e₂ = d �₂/dt

Therefore energy input to the instrument

= i₁d �₁ + i₂d �₂

= i₁d (L₁i₁ + Mi₂) + i₂d(L₂i₂ + Mi₁)

= i₁L₁di₁ + i₁²dL₁ + i₁i₂dM + i₁Mdi₂ + i₂L₂di₂ + i₂²dL₂ + i₁i₂dM + i₂Mdi₁

Since L1 and L2 are constant, therefore dL₁ = 0 and dL₂ = 0

= i₁L₁di₁ + i₁i₂dM + i₁Mdi₂ + i₂L₂di₂ + i₁i₂dM + i₂Mdi₁ ����(1)

Some of the above input energy to electrodynamometer instruments are stored in the form of magnetic energy in the coil while rest is converted into mechanical energy of moving coil. 

Thus we can write,

Energy Input = Mechanical Energy + Stored Energy

Mechanical Energy = Electrical Input � Stored Energy  ����(2)

Thus to find the mechanical energy, we need to find the change in stored energy in the magnetic field of the coil. Let us assume an infinitesimally small time dt for the sake of calculation of change in stored energy.

Change in stored energy

= d(1/2L₁i₁² + 1/2L₂i₂² + Mi₁i₂)

= i₁L₁di₁+ i₂L₂di₂+ i₁Mdi₂ + i₂Mdi₁+ i₁i₂dM+(i₁²/2)dL₁ + (i₂²/2)dL₂

But L1 and L2 are constant, therefore dL₁ = 0 and dL₂ = 0

= i₁L₁di₁+ i₂L₂di₂+ i₁Mdi₂ + i₂Mdi₁+ i₁i₂dM ��(3)

From equation (1), (2) and (3),

Mechanical Energy = i₁i₂dM

Let T_d be the dflectiong torque and dO be the change in deflection, then mechanical energy

 = T_ddO

T_ddO = i₁i₂dM

?T_d = i₁i₂dM/dO

The above equation gives the deflecting torque in electrodynamics or electrodynamometer instruments. It can be seen that deflecting torque depends upon the multiplication of instantaneous value of current and rate of change of mutual inductance between the fixed and moving coil.

Now we will consider two cases.

Case-1: When DC quantity is being measured.

Let I₁ and I₂ be the current in fixed and moving coil respectively. Therefore deflecting torque T_d = I₁I₂dM/dO

But this deflecting torque is controlled by the spring. Spring provides the controlling torque. The controlling torque due to spring for a deflection of O

Tc = KO where K is spring constant.

At equilibrium the controlling torque and deflecting torques are equal, hence

Tc = T_d

?KO = I₁I₂dM/dO

?O = (I₁I₂dM/dO)/K

Case-1: When AC quantity is being measured.

Let i1 and i2 are sinusoidal current having a phase displacement of �. Therefore we can write as

i1 = Im1Sinwt

i2 = Im2Sin(wt-�)

Thus the instantaneous deflecting torque is given as

Td = (Im1Sinwt)[ Im2Sin(wt-�)]dM/dO

The average torque for one time period of the currents are given by

Td = (I1I₂Cos�)dM/dO

Where I₁ = RMS Value of i₁

I₂ = RMS value of i₂

From the above two cases, we can have following conclusions:

• For sinusoidal alternating current, the deflecting torque is determined by the product of RMS value of coil currents and the cosine of phase angle between them.
• When the instrument is used for AC, the instantaneous torque is proportional to i². Thus the torque varies as the current varies but the direction of torque remains the same. Because of the inertia of the instrument, the needle does not follow the change in torque rather it takes a position where the average torque becomes equal to the controlling torque.

Electrodynamometer Ammeters

Figure below shows an electrodynamometer ammeter.

                                   

It can be seen that, the fixed coil and moving coil are connected in series and hence carries the same current. As the current through the moving coil shall not exceed 100 mA, therefore moving coil is shunted by suitable resistance to increase the range of such ammeter.

As the currents flowing in fixed and moving coils are same therefore there will not be any phase angle between them. Therefore, � = 0

Deflecting Torque in Ammeter

Td = I²dM/dO

where I is the RMS current flowing in fixed and moving coil.

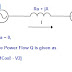
Electrodynamometer Voltmeter

Figure below shows the connection arrangement for electrodynamometer voltmeter.

In the figure above, voltmeter is connected between points A and B to measure the voltage drop across it. Note that a high value of resistance is connected in series with the coils. Why?

Since

� = 0

Therefore, Deflecting Torque in Ammeter

Td = I²dM/dO

where I is the RMS current flowing in fixed and moving coil. But I = V_AB / Z where Z is the impedance of instrument circuit. Hence,

Td =( V_AB/Z)²dM/dO

Electrodynamometer type voltmeters are the most accurate type of AC voltmeters. But the sensitivity of such instrument is low when compared from DC instruments.